16. Transforming Maxwell equations-intro and 1st equation
Maxwell's four equations:
We would like to consider how the equations need to be changed so that they apply in a lab aboard a space ship moving at velocity, V, in the x direction. The most obvious effect is that a stationary charge is now seen as moving in the negative x direction and thus being seen as a current density as well as a charge density.
We are trying to verify that the Lorentz transformations result in the same set of Maxwell's equations. Thus we will use the Lorentz transformations, as well as the transformed electric and magnetic field equations which resulted from the Lorentz transforms.
Transformation of partial derivatives
One tricky aspect of transforming Maxwell's equations from the stationary reference frame to the moving one is the need to transform the partial derivatives in those equations from using x, y, z, and t as variables into using x', y', z', and t'. In Fig. 16.1 we show the standard inverse Lorentz transform of these variables and some useful partial derivatives of these. We don't show the relationships of y and z to y' and z' since these are trivial, i.e. y' = y and z' = z and ∂y'/∂x=0, ∂y'/∂y=1, ∂y'/∂z=0, ∂y'/∂t=0, ∂z'/∂x=0, ∂z'/∂y=0, ∂z'/∂z=1, and ∂z'/∂t=0.
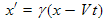
| 
| 
|

| 
| 
|
| Fig. 16.1a. Inverse Lorentz transforms of x and t. | Fig. 16.1b. Partial derivatives of the Lorentz transforms at the left. | Fig. 16.1c. More partial derivatives of the Lorentz transforms at the left. |
In Fig. 16.2 below we show the chain rule for partial derivatives applied to a typical electromagnetic field quantity, Ex in this example. We calculate the differential of Ex in terms of differential changes of the new variables x', y', z' and t'. This equation involves taking partial derivatives of Ex with respect to each of these new variables while the other new variable is held constant. One important aspect to note is which variables are held constant in the various terms.


|
| Fig. 16.2. Calculation of the differential of a typical electromagnetic field quantity Ex in terms of differentials of the variables x' and y' of the moving reference frame. |
In the next step, in Fig. 16.3, we form the partial derivative of Ex with respect to x, in the form it is found in the original Maxwell's equations, but now in terms of the partial derivatives in Fig. 16.2 above. This is one step in transforming Maxwell's equations from being written in terms of x, y, z, and t to being written in terms of x', y', z', and t'.


|
| Fig. 16.3. Calculation of the partial derivative with respect to x of a typical field quantity (Ex shown here as an example). This operation is one step in changing the variables of Ex from x, y, z, and t into x', y', z', and t', i.e. into the moving reference frame. The second equation above (boxed) is simply the first one rewritten separating out the operations from Ex. |
- The whole equation (see the left hand side) is directed towards calculating the partial of Ex with respect to x while keeping y, z, and t fixed.
But y' = y and z' = z.
Thus y' and z' are to be kept fixed and are not variables for this equation.
Thus we are only interested in the x' and t' dependence for this equation.


|
| Fig. 16.4. Calculation of the partial with respect to t. |
Transformation of the first Maxwell equation
We start out transforming the left side of the first Maxwell Equation (as shown in (16.1) above):


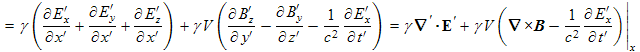
|
We next write out the transformation of the right side of the first Maxwell equation (see (16.1) above).

|
Finally, we use these in the first Maxwell equation (written with all terms moved to the left):
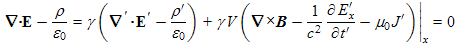
|
| Fig. 16.5. First Maxwell equation transformed to moving reference frame. |



